Simultaneous Search (with Chade, Econometrica, 5-2006)
The published paper was shrunk 50% from the much easier to read working paper here:
This paper greatly extends the classic Stigler (1961) static search paper to allow for heterogenous options. We introduce and solves portfolio choice problems such as arise when applying to college, and one may only accept one option. In our problem, the individual simultaneously chooses among ranked stochastic options, and each choice is costly. We show that a greedy algorithm that we call the marginal improvement algorithm finds the optimal set. We then characterize how this set behaves. The optimal choices are “less aggressive” than the sequentially optimal ones, but “more aggressive” than the best singletons. The optimal set in general contains gaps. We provide a comparative static on the chosen set.
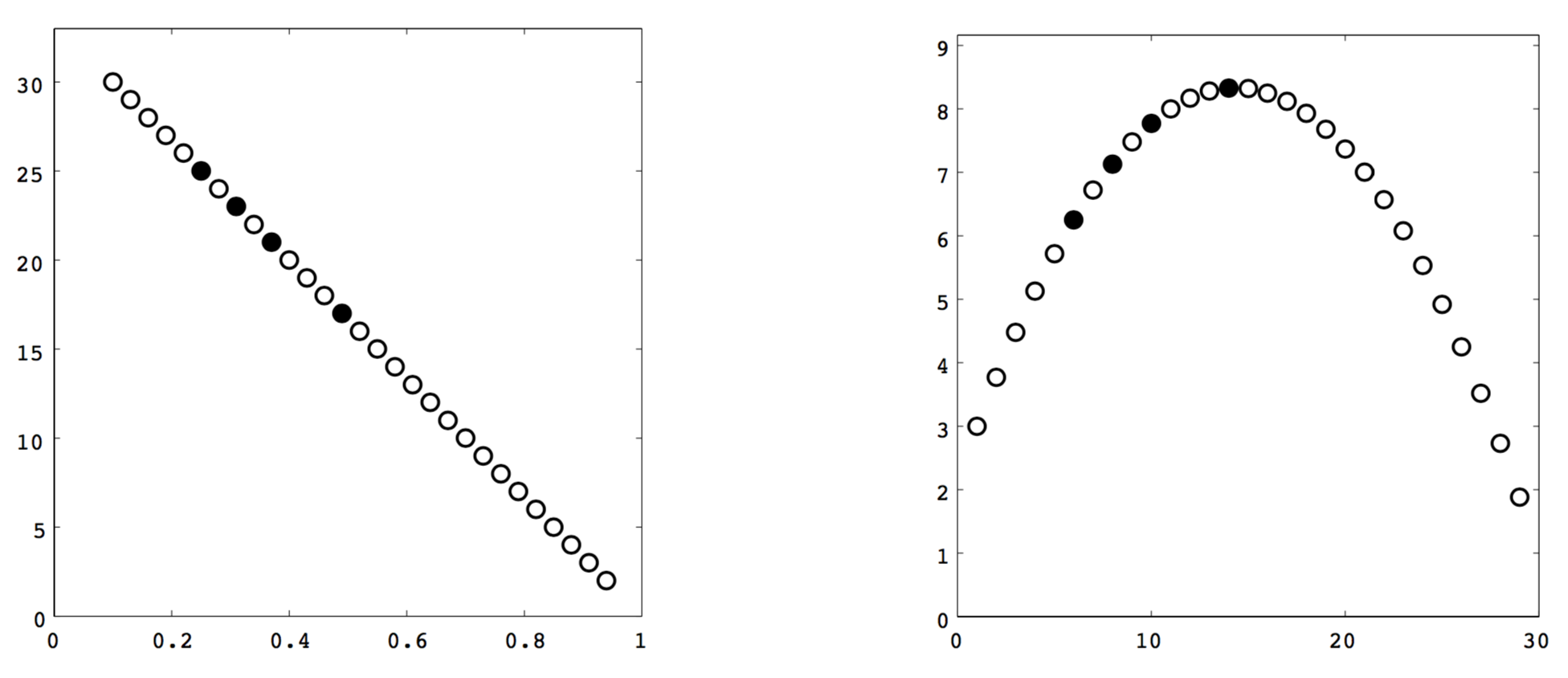
Portfolio Gambling Illustrated
The computation represents an application of our marginal improvement optimal algorithm with an application cost c = 2. At the left is the graph of college payoff u against acceptance chance α. At the right is the graph of expected college payoff z = αu against actual college payoff u. This corresponds to a student with linear payoffs u, and therefore single-peaked expected payoffs z. Chosen schools are represented by filled circles. While she does pick the best expected college, she does not exclusively choose the highest expected schools; rather, she generally gambles upward. Observe how no safety school (high expected value z, but lesser ranked) is chosen.
