“The Law of Large Demand for Information“, Moscarini and Smith (Econometrica, 2002)
Blackwell’s Theorem is the “abandon all hope” result for information value. It asserts that two signals (experiments) can only be rank ordered for all decision makers if one is a garbling of the other: to wit, the order is incredibly parial and is not subtle. We restore a complete (and somewhat subtle) order on signals by asking a slightly different question: If you could have a very large number of conditionally iid signals, which signal would you prefer? Our proof uses classical statistical result by Cramer on large deviation theory. Our result uses informational entropy to devise an intrinsic scalar measure of a signal.
But we do not stop there. A famous nonconcavity in the value of information suggests that valuing information is a lost cause, since the marginal value cannot be counted on to define an optimum. We use the very method we devise for unambiguously valuing large quantities of information to deduce an unambiguous marginal value of large quantities of information. Since it is (nearly) exponential, we deduce a (nearly) logarithmic demand for information.
Our paper applies to the modern world with extremely inexpensive information. Since demand is logarithmic, it also suggests that information sale is failing because a monopoly will never operate on the inelastic portion of his demand curve. To wit, information should be sold in large batches but at zero marginal cost.
In our companion paper, The Demand for Information: More Heat than Light, we revisit this question in a continuous Gaussian world and derive a similar answer, but now gobally valid. This highlights that this is the correct answer to the fundamental question in economics of the value of information.
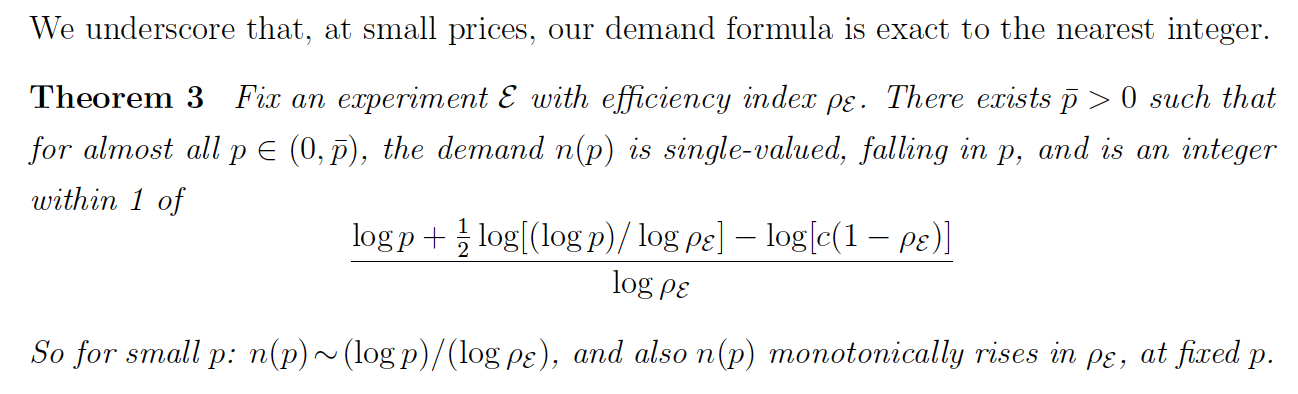
The Demand for Lots of Information is Logarithmic
Prisoner: What do you want?
Two: Information.
Prisoner: Whose side are you on?
Two: That would be telling. We want information…information…information!
